Perfect Cubes Chart
Perfect Cubes Chart - We write “n cubed” as $n^{3}$. If you can make a larger cube without any leftover little cubes, you have a perfect cube! Therefore, within the range of 1 to 100, there are precisely three perfect cubes, namely 8, 27, and 64. See a list of the first 100 perfect cubes. Web a perfect cube is defined as the product of three same integers. Includes the numbers 1 to 100 as perfect cube numbers listed in chart form and image form. The proofs for these are omitted. Thus, in other words, the cube root of x is y. To check if a number 'n' is a perfect cube or not, we check whether an integer, when multiplied by itself thrice, gives the number 'n'. But we can find cubes of any number whether it is an integer or a fraction. Includes the numbers 1 to 100 as perfect cube numbers listed in chart form and image form. See a list of the first 100 perfect cubes. For instance, the number 8 is a perfect cube because 2^3 equals 8. Web perfect squares and cubes chart number square cube 1 12 =1 13 =1 2 22 =4 23 =8 3 32. Web a perfect cube is a number, that can be written as three times the product of the same number. Web a perfect cube is defined as the product of three same integers. Web to identify the perfect cubes between 1 and 100, we search for numbers that are perfect cubes within this range. If you can make a larger. Web imagine you have a certain number of identical little cubes, and you try to assemble a larger cube with them. For example, the cube of 0.5 is: We write it as $^{3}\sqrt{x}= y$. For instance, the number 8 is a perfect cube because 2^3 equals 8. Perfect cubes can be positive or negative. Web if x is a perfect cube of y, then we represent it as $x = y^{3}$. 0.5 × 0.5 × 0.5 = 0.125. We write it as $^{3}\sqrt{x}= y$. $1^{3} = 1, 2^{3} = 8, 3^{3} = 27, 5^{3} = 125,$ etc. If you can make a larger cube without any leftover little cubes, you have a perfect cube! In this case, the perfect cubes within this range are 1, 8, 27, and 64. The perfect powers less than or equal to \(100\) are \[0,1,4,8,9,16,25,27,32,36,49,64,81,100.\] a few simple results are given below. For example, the cube of 0.5 is: Note that we can find a cube of any number, whether it is an integer or a decimal, or a.. The cube root of a perfect cube always results in a whole number. If you can make a larger cube without any leftover little cubes, you have a perfect cube! Therefore, within the range of 1 to 100, there are precisely three perfect cubes, namely 8, 27, and 64. But we can find cubes of any number whether it is. If yes, then it is a perfect cube. Web imagine you have a certain number of identical little cubes, and you try to assemble a larger cube with them. Thus, in other words, the cube root of x is y. Web the set of perfect powers is the union of the sets of perfect squares, perfect cubes, perfect fourth powers,. We write “n cubed” as $n^{3}$. If you can make a larger cube without any leftover little cubes, you have a perfect cube! In this case, the perfect cubes within this range are 1, 8, 27, and 64. Web a perfect cube is defined as the product of three same integers. Web the set of perfect powers is the union. For instance, the number 8 is a perfect cube because 2^3 equals 8. $1^{3} = 1, 2^{3} = 8, 3^{3} = 27, 5^{3} = 125,$ etc. The perfect powers less than or equal to \(100\) are \[0,1,4,8,9,16,25,27,32,36,49,64,81,100.\] a few simple results are given below. Includes the numbers 1 to 100 as perfect cube numbers listed in chart form and image. Web imagine you have a certain number of identical little cubes, and you try to assemble a larger cube with them. For example, the cube of 0.5 is: The proofs for these are omitted. Web a perfect cube is a number, that can be written as three times the product of the same number. The perfect powers less than or. Web a perfect cube is defined as the product of three same integers. We write “n cubed” as $n^{3}$. Note that we can find a cube of any number, whether it is an integer or a decimal, or a. Perfect cubes can be positive or negative. But we can find cubes of any number whether it is an integer or a fraction. $1^{3} = 1, 2^{3} = 8, 3^{3} = 27, 5^{3} = 125,$ etc. Web if x is a perfect cube of y, then we represent it as $x = y^{3}$. Web a perfect cube is a number, that can be written as three times the product of the same number. The cube root of a perfect cube always results in a whole number. We write it as $^{3}\sqrt{x}= y$. See a list of the first 100 perfect cubes. If yes, then it is a perfect cube. The proofs for these are omitted. Web to identify the perfect cubes between 1 and 100, we search for numbers that are perfect cubes within this range. Thus, in other words, the cube root of x is y. The perfect powers less than or equal to \(100\) are \[0,1,4,8,9,16,25,27,32,36,49,64,81,100.\] a few simple results are given below.
Cube. Numbers from 1 to 10 Brainly.in
![1 to 30 Cube Value [PDF Download] Cube Number](https://edukatorsclub.com/wp-content/uploads/2022/07/1-to-30-cube.jpg)
1 to 30 Cube Value [PDF Download] Cube Number
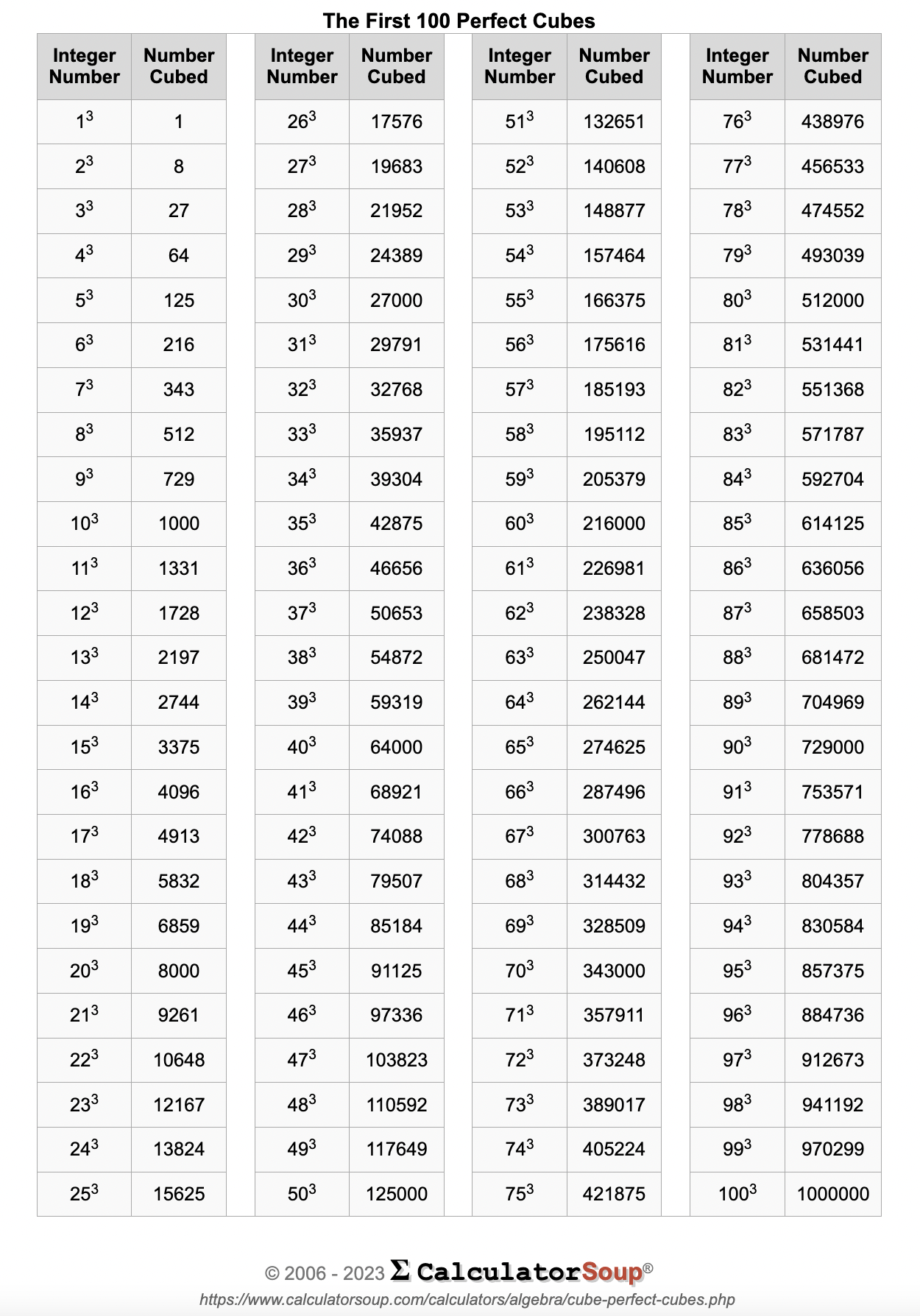
The First 100 Perfect Cubes

Identify whether the given numbers are perfect squares, perfect cubes

Perfect Cubes and Cube Roots (solutions, examples, videos, worksheets

Perfect Squares and Perfect Cubes 120 Poster Zazzle Perfect
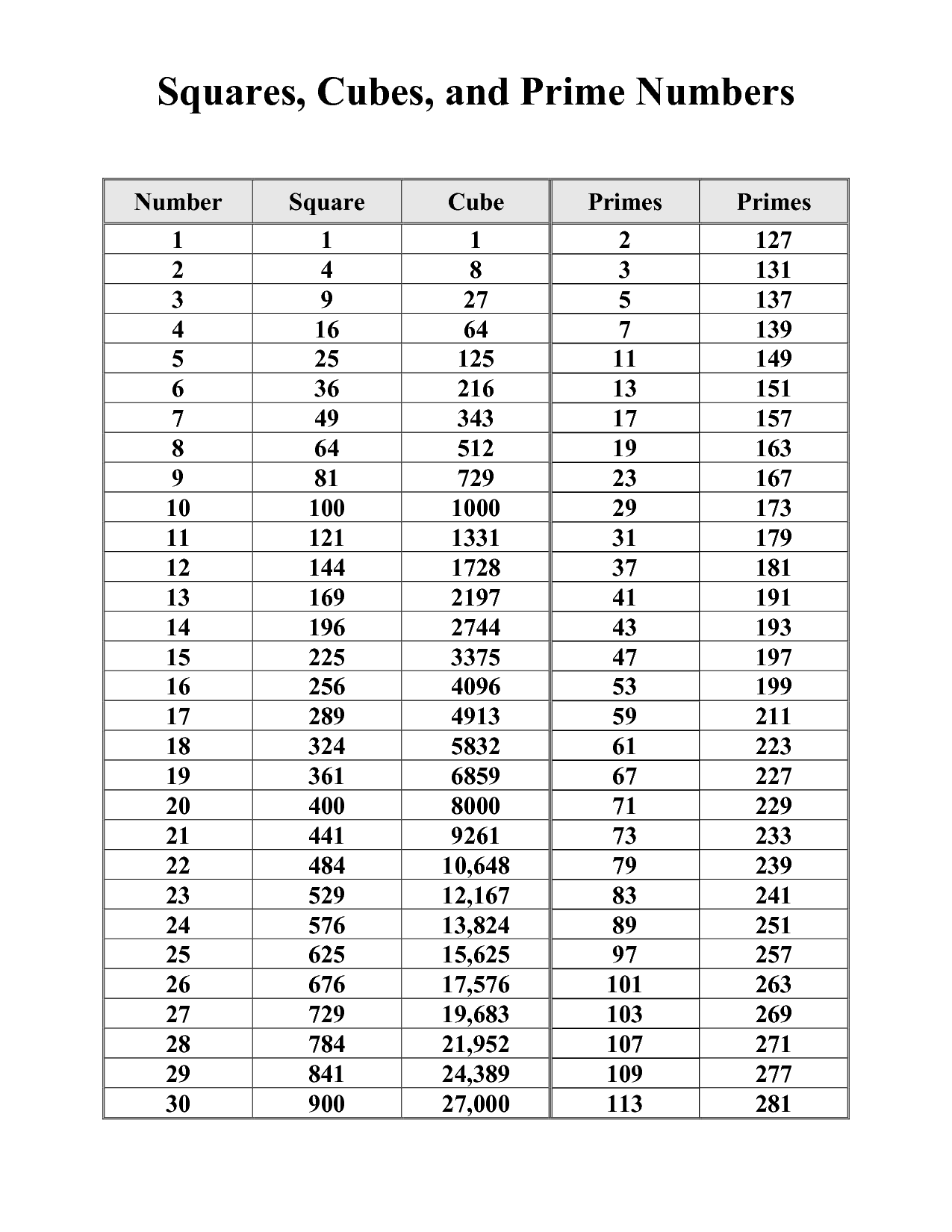
4 Best Images of Printable Number Cube Paper Cube Template, Perfect
![Cube 1 to 30 Values of Cubes from 1 to 30 [PDF Download]](https://wmznlejcfq.s3-ap-southeast-1.amazonaws.com/media/square-roots/cubes-1-to-30.png)
Cube 1 to 30 Values of Cubes from 1 to 30 [PDF Download]

Perfect Cube Series Tricks Examlogger IAS/IPS, SSC/Bank Exam tips
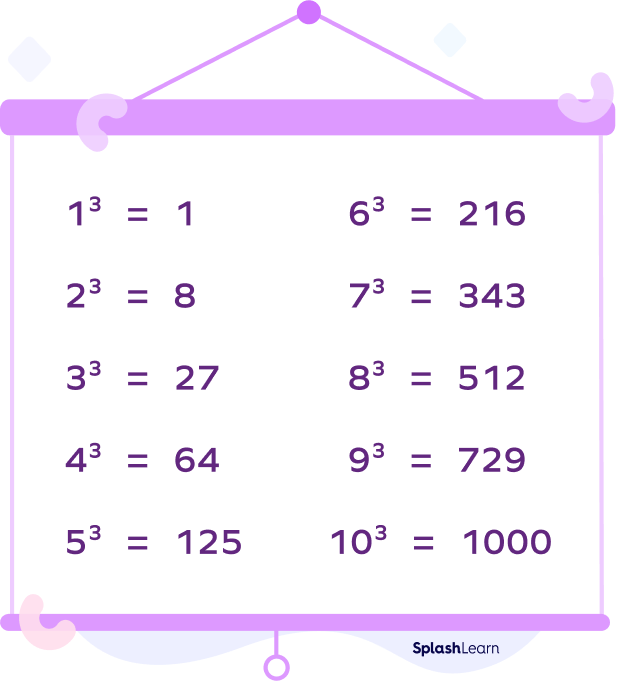
Perfect Cube of Numbers Definition, List, Chart, Examples, Fact
0.5 × 0.5 × 0.5 = 0.125.
Therefore, Within The Range Of 1 To 100, There Are Precisely Three Perfect Cubes, Namely 8, 27, And 64.
If You Can Make A Larger Cube Without Any Leftover Little Cubes, You Have A Perfect Cube!
To Check If A Number 'N' Is A Perfect Cube Or Not, We Check Whether An Integer, When Multiplied By Itself Thrice, Gives The Number 'N'.
Related Post: